Group B, Poster #194, Earthquake Forecasting and Predictability (EFP)
How epistemic uncertainty of sub-surface fault geometry affects large earthquakes recurrence variability
Poster Image:
Poster Presentation
2022 SCEC Annual Meeting, Poster #194, SCEC Contribution #11889 VIEW PDF
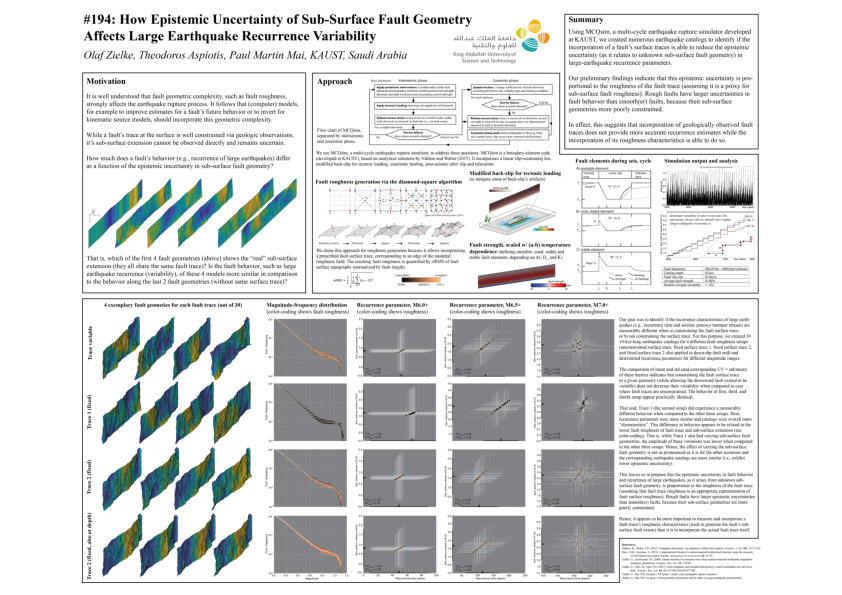
The geometric complexity of fault surfaces (i.e., their fault roughness) strongly influences seismotectonic behavior, affecting the initiation, propagation, and termination of individual earthquakes as well as the stress-slip relationship, fault segmentation, and probability of multi-segment rupture. Consequently, computer simulations that aim to resolve the earthquake rupture process and make predictions of a fault’s future behavior should (and often do) incorporate fault geometric complexity. However, doing so exhibits some inherent difficulties: Although we may be able to constrain a fault’s geometry with a high level of detail at the ground surface (e.g., documenting a fault trace at (deca-) meter resolution), we cannot do the same for the buried portion of the fault. Most of a fault’s geometry remains unconstrained, raising the question how relevant the incorporation of high-resolution fault trace geometry in computer simulations actually is. That is, how much does the detailed implementation of a fault trace affect the epistemic uncertainty in a fault’s seismotectonic behavior, given that the majority of its geometry remains unconstrained?
We address this question computationally with a physics-based, multi-cycle earthquake rupture simulator (MCQsim) that enables us to investigate how (for example) earthquake recurrence, slip accumulation, magnitude-frequency distribution, and fault segmentation vary as function of our insufficient knowledge about the fault’s down-dip geometric complexity. We incorporate fault geometric complexity using the “random midpoint displacement” method. This method permits creating self-affine, 2-D random fields while simultaneously keeping the random field’s edges (e.g., the surface trace) fixed. We create 100+ seismic catalogs (each 10-kyr long, for all primary faulting styles) along predefined fault surfaces (and fixed fault traces) to investigate how earthquake recurrence (i.e., its variability) and other seismotectonic parameters are affected by fault geometric uncertainty. In the process, we find for example that the variability in earthquake recurrence time (and corresponding slip increment) is not significantly reduced when the fault's surface trace is implemented (relative to a scenario where it is not considered). Implementing the fault's surface trace at high resolution appears to not improve our ability to forecast the fault's future behavior.
We address this question computationally with a physics-based, multi-cycle earthquake rupture simulator (MCQsim) that enables us to investigate how (for example) earthquake recurrence, slip accumulation, magnitude-frequency distribution, and fault segmentation vary as function of our insufficient knowledge about the fault’s down-dip geometric complexity. We incorporate fault geometric complexity using the “random midpoint displacement” method. This method permits creating self-affine, 2-D random fields while simultaneously keeping the random field’s edges (e.g., the surface trace) fixed. We create 100+ seismic catalogs (each 10-kyr long, for all primary faulting styles) along predefined fault surfaces (and fixed fault traces) to investigate how earthquake recurrence (i.e., its variability) and other seismotectonic parameters are affected by fault geometric uncertainty. In the process, we find for example that the variability in earthquake recurrence time (and corresponding slip increment) is not significantly reduced when the fault's surface trace is implemented (relative to a scenario where it is not considered). Implementing the fault's surface trace at high resolution appears to not improve our ability to forecast the fault's future behavior.



















